Shape and Space
Symmetry:
- The quality of being made up of exactly similar parts facing each other or around an axis.
- Exact correspondence of form and constituent configuration on opposite sides of a dividing line or plane or about a center or an axis.
Length
Various instruments are used to measure length. For example:
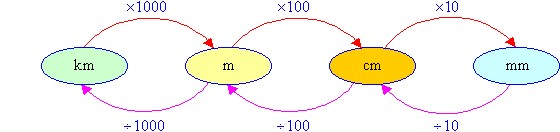
To convert length from a larger unit into a smaller unit, multiply by the relevant power of 10.
To convert length from a smaller unit into a larger unit, divide by the relevant power of 10.
Example.
Convert the following measurements to the units indicated:
a. 7 cm to mm
b. 8 m to cm
c. 9 km to m
Solution:
- Rulers and tape measures are marked in millimetres or centimetres to measure shorter lengths accurately.
- A trundle wheel is used to measure length to the nearest metre. Note that a counter may be attached to the trundle wheel to count metres.
- A car's odometer often measures distance in tenths of a kilometre.
To convert length from a larger unit into a smaller unit, multiply by the relevant power of 10.
To convert length from a smaller unit into a larger unit, divide by the relevant power of 10.
Example.
Convert the following measurements to the units indicated:
a. 7 cm to mm
b. 8 m to cm
c. 9 km to m
Solution:
Whole Number: Division
- In mathematics, especially in elementary arithmetic, division (÷) is an arithmetic operation.
- Another basic view of division is sharing equally.
Example:
Danesse has 3 friends. She has 12 cookies to share with them. How many cookies does each of the four people get?
- Danesse probably shared her cookies by saying one for you, one for you, so on. Thus the 12 cookies are shared equally by four people, and each gets three cookies.
- Or, you might say that the 12 cookies have been partitioned into four sets of the same size.
Exercises:
- 21 ÷ 3 = ________
- 24 ÷ 4 = ________
- 42 ÷ 7 = ________
- 32 ÷ 8 = ________
- 24 ÷ 8 = ________
- 54 ÷ 6 = ________
- 72 ÷ 6 = ________
- 27 ÷ 9 = ________
- 72 ÷ 9 = ________
- 56 ÷ 7 = ________
Fractions
- A fraction is a number that is written in the form:
- The a is the numerator, and the b is the denominator.
- The line separating the numerator and denominator is a fraction bar.
- Fractions are used when representing numbers that describe the parts of a whole. The fraction a/b also can be read as "a out of b" ,"a over b" or "a divided by b."
- Both a and b must be integers, meaning positive and negative whole numbers.
- The denominator, or b, cannot be zero. This is because one cannot divide by zero.
Example:
If there are 18 students in a classroom, and 6 of the students wear glasses, what fraction of the students wear glasses?
- A fraction can be thought of as "a out of b."
- Total number of students is 18.
- Number of students with glasses is 6.
- Number of students with glasses out of the whole class is 6/18.
Whole Numbers: Multiplication
- Multiplication (often denoted by the cross symbol "×") is the mathematical operation of scaling one number by another.
- Whole-number products greater than 1 can be computed by repeated addition;
- 7 multiplied by 3 (often said as "7 times 3") can be calculated by adding 7 copies of 3 together: 7 x 3 = 3 + 3 + 3 + 3 + 3 + 3 + 3 = 21
- Here 7 and 3 are the "factors" and 21 is the "product".
- 7 multiplied by 3 can also be calculated by adding 3 copies of 7 together: 7 x 3 = 7 + 7 + 7 = 21
Exercises:
- 7 x 1 =
- 6 x 5 =
- 7 x 8 =
- 3 x 9 =
- 6 x 6 =
- 9 x 8 =
- 5 x 7 =
- 6 x 9 =
- 2 x 8 =
- 9 x 9 =